Selected Works

1. A self-organized critical model of dynamical hydrogen-bonded network (In preparation)

Author: Tridib Sadhu

We consider a toy model of rearranging hydrogen bonded network in water which shows self-organized critical behavior. The model is defined on a $L\times M$ square lattice with open boundary. Each lattice site is occupied by a water molecule with its oxygen atom sitting on the lattice vertices and two hydrogen atoms along the lattice edges with their bonds at right angle to other. The model satisfies standard ice constraint that any bond occupied by two hydrogen atom costs energy.

We define a discrete time dynamics such that the model in any excited state would relax to its ground state following a set of relaxation step at every site. The model, slowly driven by a forced rotation of a randomly chosen water molecule, would jump from one ground state to other. The steady state of this model exhibits power law distribution of avalanche sizes. The model is non-Abelian and energy is not conserved in an avalanche. We show that there are exactly $\left( L+1 \right)^{M}\left( M+1 \right)^{L}$ recurrent configurations. We determine the steady state probability of the model on a $L\times1$ lattice and determine the distribution of avalanche sizes. For the two dimensional model we show that the distribution can be described by a linear combination of two simple scaling forms.

Post Info - Category | 0 Comments

2. Non-compact growth of patterns in the sandpile models at the edge of stability (In preparation)

Authors: Tridib Sadhu and Prof. Deepak Dhar

Feeding sand grains at a single site in the sandpile models of self-organized criticality triggers avalanches whose size depends on the initial height distribution (background). For some backgrounds avalanches of infinite length will occur with absolute certainty. For those where the avalanches are always finite, the affected region forms an asymptotic pattern. In all the examples studied so far, the patterns grow as $N^{1/d}$, with $N$ being the number of grains added and $d$ being the spatial dimension of the lattice. There are backgrounds where the growth is in the intermediate regime: All the avalanches are finite but the growth is faster than $N^{1/d}$. For stochastic models such backgrounds are sharply characterized in terms of a critical density of heights. However in a deterministic model such a critical value is not defined and it is even nontrivial to find one such background. We discovered one such background for a deterministic sandpile model on a directed triangular lattice, where the pattern grows linearly with $N$. This is the simplest, yet nontrivial pattern with proportionate growth, studied so far and we give a complete analytical characterization of the pattern.

We also discuss a deterministic algorithm for generating complex patterns using discrete approximants of continuous functions.

Post Info - Category | 0 Comments

3. Pattern Formation in Growing Sandpiles with Multiple Sources and Sinks

Authors: Tridib Sadhu and Prof. Deepak Dhar

Adding sand grains at a single site in Abelian sandpile models produces beautiful but complex patterns. We study the effect of sink sites on such patterns. Sinks change the scaling of the diameter of the pattern with the number $N$ of sand grains added. For example, in two dimensions, in presence of a sink site, the diameter of the pattern grows as $\sqrt{(N/\log N)}$ for large $N$, whereas it grows as $\sqrt{N}$ if there are no sink sites. In presence of a line of sink sites, this rate reduces to $N^{1/3}$. We determine the growth rates for these sink geometries along with the case when there are two lines of sink sites forming a wedge, and its generalization to higher dimensions.

We characterize one such asymptotic patterns on the two-dimensional F-lattice with a single source adjacent to a line of sink sites, in terms of position of different spatial features in the pattern. For this lattice, we also provide an exact characterization of the pattern with two sources, when the line joining them is along one of the axes.

Post Info - Category | 0 Comments

4. Steady State of Stochastic Sandpile Models

Authors: Tridib Sadhu and Prof. Deepak Dhar

We study the steady state of the Abelian sandpile models with stochastic toppling rules. The particle addition operators commute with each other, but in general these operators need not be diagonalizable. We use their Abelian algebra to determine their eigenvalues, and the Jordan block structure. These are then used to determine the probability of different configurations in the steady state. We illustrate this procedure by explicitly determining the numerically exact steady state for a one dimensional example, for systems of size ≤12, and also study the density profile in the steady state.

Post Info - Category | 0 Comments

5. Pattern Formation in Growing Sandpiles

Authors: Prof. Deepak Dhar, Tridib Sadhu and Samarth Chandra

Adding grains at a single site on a flat substrate in the Abelian sandpile models produces beautiful complex patterns. We study in detail the pattern produced by adding grains on a two-dimensional square lattice with directed edges (each site has two arrows directed inward and two outward), starting with a periodic background with half the sites occupied. The model shows proportionate growth and the size of the pattern formed by adding N grains scales as $\sqrt{N} $. We give exact characterization of the asymptotic pattern, in terms of the position and shape of different features of the pattern.

Post Info - Category | 0 Comments

6. Emergence of quasiunits in the one-dimensional Zhang model

Authors: Tridib Sadhu and Prof. Deepak Dhar

We study the Zhang model of sandpile on a one-dimensional chain of length L, where a random amount of energy is added at a randomly chosen site at each time step. We show that in spite of this randomness in the input energy, the probability distribution function of energy at a site in the steady state is sharply peaked, and the width of the peak decreases as L−1/2 for large L. We discuss how the energy added at one time is distributed among different sites by topplings with time. We relate this distribution to the time-dependent probability distribution of the position of a marked grain in the one-dimensional Abelian model with discrete heights. We argue that in the large L limit, the variance of energy at site x has a scaling form L−1g(x/L), where g(xi) varies as ln(1/xi) for small xi, which agrees very well with the results from numerical simulations.

Post Info - Category | 0 Comments

7. Identity configurations in sandpile models (Unpublished)

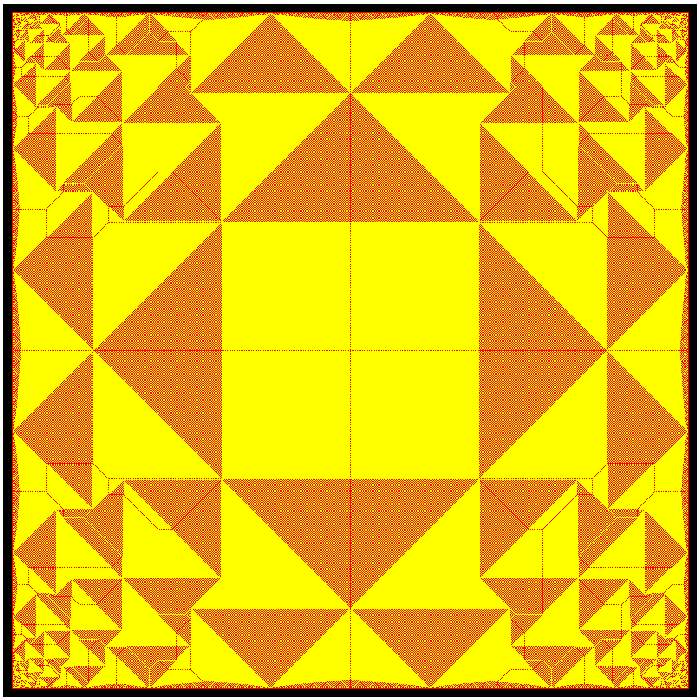
One of the Identity configurations for a sandpile model on a directed variant of square lattice is characterized by Caracciolo et. al.. We provide an alternative approach of characterizing such patterns.

Post Info - Category | 0 Comments